書裡還有很多值得準備的題目,google books有的我就將網址列出來
沒有的我就將內容抄出來,想知道更多題目可以到圖書館去找這本書。
------------------------------
Prove that the number
c=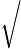 391+
391+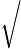 3−92+
3−92+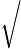 394
394 is a zero of
F(x)=x3+ 36x2−1
36x2−1 .
第44頁
http://books.google.com.tw/books?id=l0CDp-YVyjoC&lpg=PP1&ots=01zdioOshi&dq=Equations%20and%20Inequalities:Elementary%20Problems%20and%20Theorems%20in%20Algebra%20and%20Number%20Theory&pg=PA44#v=onepage&q&f=true
求滿足下列等式的數a:
 3
3 32−1=1
32−1=1 3a(1−
3a(1− 32+
32+ 34)
34)
(1995日本奧林匹克預選賽)
若
 3
3 32−1=
32−1= 3a+
3a+ 3b+
3b+ 3c
3c ,則
a+b+c=?
(92高中數學能力競賽,高中數學101 P25)
110.9.23補充
 3
3 32−1=
32−1= 3a+
3a+ 3b+
3b+ 3c
3c ,其中
a、
b、
c Q
Q。求
a+b+c= 。
(100中科實中,
https://math.pro/db/viewthread.php?tid=1107&page=3#pid3377)
112.8.21補充
已知實數
x滿足拉馬努金等式
 3
3 32−1=x1−
32−1=x1− 32+
32+ 34
34 ,求實數
x的值。(須以最簡單形式表示)
(111高中數學能力競賽 第一區(花蓮高中)口試試題,
https://math.pro/db/thread-3782-1-1.html)
當
 3
3 316−2=
316−2= 3a+
3a+ 3b+
3b+ 3c
3c ,其中
a b
b c
c Q
Q。求
a+b+c。
(109第1學期中山大學雙週一題)
110.2.25補充
若
a是一個有理數且滿足
1 34+
34+ 32+a=
32+a=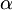
 34+
34+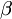
 32+
32+
,其中
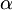

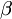


為有理數。試求
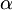

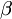


(用
a表示)
(109北科附工,
https://math.pro/db/viewthread.php?tid=3326&page=2#pid21223)
111.1.10補充
有理化
1 32+
32+ 33+
33+ 35
35
------------------------------
For the expression
Q(n)=21 43
43 65
65

 2n2n−1
2n2n−1 (
n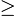 2
2 )
we will prove the bounds
12 n
n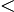 Q(n)
Q(n)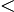 1
1 2n+1
2n+1.
第103頁
http://books.google.com.tw/books?id=l0CDp-YVyjoC&lpg=PP1&ots=01zdioOshi&dq=Equations%20and%20Inequalities:Elementary%20Problems%20and%20Theorems%20in%20Algebra%20and%20Number%20Theory&pg=PA103#v=onepage&q&f=true
an=2 4
4 6
6

 (2n)1
(2n)1 3
3 5
5

 (2n−1)
(2n−1),試求
limn
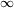 an
an。
(97文華高中,連結已失效h ttp://forum.nta.org.tw/examservice/showthread.php?t=47781)
------------------------------
Show that for arbitary
x y
y z
z R
R we have
(x2+y3+z6)2 2x2+3y2+6z2
2x2+3y2+6z2 (43)
Furthermore,determine when equality occures in (43).
第129頁
http://books.google.com.tw/books?id=l0CDp-YVyjoC&lpg=PP1&ots=01zdioOshi&dq=Equations%20and%20Inequalities:Elementary%20Problems%20and%20Theorems%20in%20Algebra%20and%20Number%20Theory&pg=PA129#v=onepage&q&f=true
求
(x2+y3+z6)2=2x2+3y2+6z2的所有整數解。
(90高中數學能力競賽 中彰投區試題)
h ttp://www.math.nuk.edu.tw/senpengeu/HighSchool/2002_Taiwan_High_Taichung_02.pdf 連結已失效
------------------------------
Show that the inequality
 x+1+
x+1+ 2x−3+
2x−3+ 50−3x
50−3x 12
12 holds for all values
x R
R for which the left-hand side is defined.
SOLUTION. By (48) with
n=3,
u1= x+1
x+1 ,
u2= 2x−3
2x−3 , and
u3= 50−3x
50−3x
we have
( x+1+
x+1+ 2x−3+
2x−3+ 50−3x)2
50−3x)2 3(x+1+2x−3+50−3x)=144
3(x+1+2x−3+50−3x)=144 ,
from which, upon taking the square root, we obtain the result.
求函數
y= x+27+
x+27+ 13−x+
13−x+ x
x 的最大和最小值。
(2009大陸高中數學競賽)
------------------------------
Given an aribitrary prime p, solve the Diophantine equation
x4+4x=p.(第245頁)
SOLUTION. For any integer
x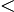 0
0,
4x is not an integer, and neither is
x4+4x.
Thus for any prime p, the given equation has no negative solution.
For
x=0 we have
04+40=1, which is not a prime, and for
x=1 we have
14+41=5, a prime.
We will now show that for any integer
x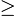 2
2, the number
x4+4x is composite. If
x=2k is even, where
k N
N, then
x4+4x=24k4+42k=16(k4+42(k−1)),
which is a composite number. If
x=2k+1(
k N
N ) is odd, then
x4+4x=x4+4 42k=[x4+4x2(2k)2+4(2k)4]−4x2(2k)2
=[x2+2(2k)2]2−(2x
42k=[x4+4x2(2k)2+4(2k)4]−4x2(2k)2
=[x2+2(2k)2]2−(2x 2k)2
=[x2+2x
2k)2
=[x2+2x 2k+2(2k)2][x2−2x
2k+2(2k)2][x2−2x 2k+2(2k)2]
=[(x+2k)2+22k][(x−2k)2+22k]
2k+2(2k)2]
=[(x+2k)2+22k][(x−2k)2+22k],
which is again compostie, since
(x±2k)2+22k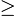 22k
22k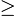 22
22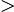 1
1.
To summarize, for
p=5 the given equation has the unique solution
x=1 ,while there are no solutions for any prime
p \ne 5
Prove that if
n>1 then
n^4+4^n is compostie.
http://www.artofproblemsolving.com/Forum/viewtopic.php?t=328143
設
n,a 為自然數,試證:有無限多個a使得
n^4+a 必不為質數
(藍藍天上一朵雲 歷屆教甄考題整理2005版第75題)
Prove that there are infinitely many natural numbers a with the following property: the number
z=n^4+a is not prime for any natural number n.
(1969IMO,
http://www.artofproblemsolving.c ... p/1969_IMO_Problems)
Compute
\displaystyle \frac{(10^4+324)(22^4+324)(34^4+324)(46^4+324)(58^4+324)}{(4^4+324)(16^4+324)(28^4+324)(40^4+324)(52^4+324)} .
(1987AIME)
101.1.10補充
100卓蘭實驗高中,
https://math.pro/db/thread-1165-1-1.html
高中數學101 P26