填 10. 補充類題
再來一個
amc 2012
Let
f(x)=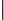 2
2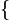 x
x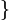 −1
−1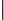
where
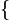 x
x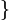
denotes the fractional part of
x . The number
n is the smallest pohsitive integer such that the equation
nf(xf(x))=2012 has at least 2012 real solutions
x. What is
n ?
Note: the fractional part of
x is a real number
y=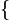 x
x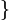
, such that
0 y
y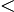 1
1 and
x−y is an integer.