已知
x+y+z=2，
x2+y2+z2=3，
x3+x3+z3=4，試求
x4+x4+z4。
(我的教甄準備之路 利用根與係數的關係解聯立方程式)
https://math.pro/db/viewthread.php?tid=661&page=1#pid1076
已知
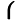
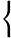
a+b=8ax+by=9ax2+by2=57ax3+by3=111，求
ax4+by4
補充一題
設c,d,x,y為實數,滿足
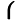
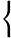
ax+by=3ax2+by2=7ax3+by3=16ax4+by4=42，求
cx5+dy5之值
連結已失效h ttp://forum.nta.org.tw/examservice/showthread.php?t=47266
連結已失效h ttp://forum.nta.org.tw/examservice/showthread.php?t=26666
Find
ax5+by5 if the real numbers
a
bxand
y satisfy the equations
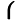
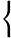
ax+by=3ax2+by2=7ax3+by3=16ax4+by4=42
(1990AIME，
https://artofproblemsolving.com/ ... Problems/Problem_15
99鳳新高中，
https://math.pro/db/thread-974-1-1.html)
這裡補充用遞迴的方法
令
An=axn+byn
axn+1+byn+1=(x+y)(axn+byn)−xy(axn−1+byn−1)
111=(x+y)(57)−xy(9)57=(x+y)(9)−xy(8)
得
x+y=1，
xy=−6，
An+1=An+6An−1
ax4+by4=(ax3+by3)+6(ax2+by2)=453
2009.9.27補充
http://www.yll.url.tw/viewtopic.php?t=23885
2010.3.27補充
98學年度第二學期中山大學雙週一題
http://www.math.nsysu.edu.tw/~problem/2010s/2Q.pdf
2010.3.29補充
第3,4題
http://www.shiner.idv.tw/teachers/viewtopic.php?p=3014
設實數c,d,x,y滿足
cx+dy=3，
cx3+dy3=16且
cx2+dy2=3，
cx4+dy4=16試求：
cx5+dy5之值。
(94高中數學能力競賽 高屏區筆試一)
2010.6.27補充
The sum of three numbers is 6, the sum of their squares is 8, and the sum of their cubes is 5. What is the sum of their fourth powers?
連結已失效h ttp://www.artofproblemsolving.com/Forum/viewtopic.php?f=150&t=354265
101.6.28補充
若
abxy
R，
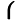
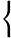
a+b=4ax+by=13ax2+by2=41ax3+by3=127，求
ax4+by4
(101中正高中二招，
https://math.pro/db/thread-1446-1-1.html)
103.5.15補充
若
a+b=1ax+by=−1ax2+by2=−5ax3+by3=−13，求
ax5+by5之值為
。
(103彰化高中，
https://math.pro/db/thread-1890-1-1.html)
104.5.2補充
已知實數
xyab滿足
ax+by=1ax2+by2=2ax3+by3=8ax5+by5=100，則
ax4+by4= 。
(104鳳山高中，
https://math.pro/db/thread-2244-1-1.html)
