第5題：

原式整理得 log12(x+4x)=log34x 

設 k=log34x     4x=3k  且 x=9k 

原式變成 log12(9k+3k)=k    9k+3k=12k

912k+312k=1     k=1    x=81

類似題可參考101松商 https://math.pro/db/viewthread.php?tid=1425&page=2#pid6736

證明2：

剛剛才想到可以利用方向餘弦的概念來證明！

cos2+cos2+cos2=1 表示 (coscoscos) 形成一組方向餘弦

故可設 (coscoscos)=xx2+y2+z2yx2+y2+z2zx2+y2+z2   (三個角度皆銳角，故此地 xyz0)

tan+tan+tan=xy2+z2+yz2+x2+zx2+y2 

                                        x2yz+y2zx+z2xy233xyzxyz=32 

兩個不等式都是由算幾不等式得到！

[ 本帖最後由 Pacers31 於 2014-4-29 08:51 AM 編輯 ]

各種排列出現的機率不相等，我們用縮小數字比較方便指出不相等之處

假設 6 支籤，2支有獎，4支沒獎，甲乙丙依序各 2 支籤，但甲、乙只要抽中獎之後，(如果還有另一支沒抽)另一支就強制為不中獎的籤。
就是這個設定，讓甲的第1, 2 支在排列中失去相同的地位了，使得第一支籤比第二支容易中獎

我們看看機率，甲中獎情形有：(1) 1中2不中，機率為 31 (2) 1不中2中，機率為 64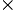52=415

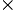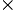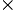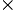更直接一點來看兩種排法 oxoxxx 和 xooxxx (o表示中獎)，各別的機率為 62141 和 645241

[ 本帖最後由 tsusy 於 2014-6-7 11:46 PM 編輯 ]

填充8. 97 台中二中曾考過類似題，看完 Elllipse 兄的神解之後，不妨做看看

97 台中二中計算10. 若 Z=1 且滿足 Z28−Z8−1=0 的複數共有 n 個，分別為 zk=cosk+isink，其中 0123n360，
則 (1) n=?   (2) 求 1+3+5++n−1

答. (1) 8  (2) 310

[ 本帖最後由 tsusy 於 2014-4-29 12:23 PM 編輯 ]

引用:

原帖由 tsusy 於 2014-4-29 12:21 PM 發表
填充8. 97 台中二中曾考過類似題，看完 Elllipse 兄的神解之後，不妨做看看

97 台中二中計算10. 若 Z=1 且滿足 Z28−Z8−1=0 的複數共有 n 個，分別為 \( z_k = \cos \theta_k + i \sin \the ...

請問是否解出來的z還要帶回原來題目驗證? 因為97台中一中的還需要帶回原來的方程式去驗算是否滿足方程式?謝謝!

請問填充第7題，小弟的做法不知道可以嗎？有更好的做法嗎？

1.
an+an+1=cnanan+1=15n
2.
a1=2，a2=110，a3=52，a4=150，…
奇數項公比=51，偶數項公比=51
3.
S2n=2nk=1ck=2nk=1(ak+ak+1)=2nk=1ak+2nk=1ak+1 
其中2nk=1ak=1−5121−(51)n+1−511101−(51)n 

2nk=1ak+1=a1+a2+a3++a2n−1−a1=1−5121−51n+1+1−511101−51n−2 

∴limnS2n=25+81+25+81−2=413

想請教填充3 &6&16題

引用:

原帖由 sorze 於 2014-4-28 10:41 PM 發表
第9題
甲乙丙中獎機率皆為2/3 未中獎1/3
三種情形 甲 乙 丙
               O  X  O
               X  O  O
               X  X  O
所求=[(2/3)(1/3)(2/3)] / [(2/3)(1/3)(2/3)+(1/3)(2/3)(2/3)+(1/3)(1/3)(2/3)] ...

請問老師
甲乙丙中獎機率 為什麼 皆為2/3 ?
謝謝

引用:

原帖由 panda.xiong 於 2014-5-1 08:34 AM 發表
請問填充第4題，小弟的做法不知道可以嗎？有更好的做法嗎？

後半段算 2(a1+a2+a3+a4+................) -a1
就可以了~

想問有人有算12題嗎?我怎麼模仿算出的是75@@。

引用:

原帖由 bugmens 於 2014-4-26 01:26 PM 發表
6.
若secx+tanx=722，則cscx+cotx之值為？

Suppose that secx+tanx=722 and that cscx+cotx=nm, where \( \displaysty ...

因為這想法是錯的~
寸絲老師已經在32樓回覆了