6.
一個實係數三次多項式函數通過
(101
2012)、
(992008)、
(1022005)、
(1032016)四點，求此函數的切線中，斜率最小的切線所在的直線方程式為？
[解法]
可以用這篇所提到的牛頓差值多項式來解題
https://math.pro/db/viewthread.php?tid=661&page=2#pid5274
將這四點向左平移99，向下平移2008
f(0)0yf(1)y4−2y4−y−15+3yf(2)4y−11−729−yf(3)−318 11f(4)8
三次多項式在三階差分時會相等
−15+3y=29−y，
y=11
f(n)=0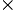
C0n+11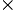C1n−18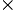C2n+18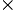C3n=3n3−18n2+26n
f(x)=3x3−18x2+26x
f
(x)=9x2−36x+26=9(x−2)2−10
過點
(24)有最小斜率-10
平移回去
過點
(1012012)有最小斜率-10
切線方程式為
y−2012=−10(x−101)，
10x+y=3022
9.
有一組正整數
a2，
a3，
a4，
a5，
a6，
a7使得
74=2!a2+3!a3+4!a4+5!a5+6!a6+7!a7，其中
0
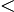ai
i(i=2,3,4,5,6,7)，求數對
(a2a3a4a5a6a7)
有唯一一組整數
a2，
a3，
a4，
a5，
a6，
a7使得
74=2!a2+3!a3+4!a4+5!a5+6!a6+7!a7，其中
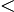0aii(i=2,3,4,5,6,7)，求
a2+a3+a4+a5+a6+a7=？
(A)8 (B)9 (C)10 (D)11
(97台南縣國中聯招，h ttp://forum.nta.org.tw/examservice/showthread.php?t=50888 連結已失效)
There are unique integers
a_2,a_3,a_4,a_5,a_6,a_7 such that
\displaystyle \frac{5}{7}=\frac{a_2}{2!}+\frac{a_3}{3!}+\frac{a_4}{4!}+\frac{a_5}{5!}+\frac{a_6}{6!}+\frac{a_7}{7!} , where
0 \le a_i < i for i=2,3,4,5,6,7. Find
a_2+a_3+a_4+a_5+a_6+a_7 .
(A)8 (B)9 (C)10 (D)11 (E)12
(1999AMC12，
http://www.artofproblemsolving.c ... 82&cid=44&year=1999)
112.6.17補充
若
n為正整數，定義
n!(讀作
n的階乘)為從1到
n的所有正整數之蓮乘積，即
n!=1\cdot 2\cdot 3\ldots n，設
0\le a_k<k，其中
a_k為整數，已知
\displaystyle \frac{a_2}{2!}+\frac{a_3}{3!}+\frac{a_4}{4!}+\frac{a_5}{5!}+\frac{a_6}{6!}+\frac{a_7}{7!}=\frac{4}{7} ，求
a_2+a_3+a_4+a_5+a_6+a_7之值。
(建中通訊解題第155期，
http://web2.ck.tp.edu.tw/~mathwe ... 30-15&Itemid=37)
10.
設甲、乙兩袋中，甲袋有1白球1黑球，乙袋有1白球，從甲袋隨機取1球放入乙袋後，再從乙袋隨機取1球放回甲袋，完成這樣的動作稱為一局，試求
n局後甲袋有1白球1黑球的機率？(答案以
n表示)
(105彰化高中，
https://math.pro/db/thread-2492-1-1.html)
(110彰化女中，
https://math.pro/db/thread-3514-1-1.html)
11.
實數a,b滿足
(a+bi)^{101}=a-bi (其中
i=\sqrt{-1} )，則數對
(a,b) 有組解
Find the number of ordered pairs of real numbers
(a,b) such that
(a+bi)^{2002}=a-bi .
(A)1001 (B)1002 (C)2001 (D)2002 (E)2004
(2002AMC12，
https://artofproblemsolving.com/ ... Problems/Problem_24)
13.
將十次多項式
(x+1)(x+2)(x+3)(x+4)(x+5)(x+6)(x+7)(x+8)(x+9)(x+10) 展開後得
x^{10}+55x^9+a_8x^8+a_7x^7+...+10! ，若
a_8=55M ，
a_7=55^2 N ，其中M、N為正整數，求數對
(M,N)= ？
thepiano所提供的解法
http://www.shiner.idv.tw/teachers/viewtopic.php?f=53&p=7448#p7437
但這個公式是我在2008年在ptt數學版看到的，想不到過了這麼多年這篇文章終於派上用場，請參閱附加檔案
15.
四邊形ABCD，
\overline{AB}=14 、
\overline{BC}=9 、
\overline{CD}=7 、
\overline{DA}=12 ，求四邊形ABCD的所有內切圓中，面積最大者為
Consider all quadrilaterals ABCD such that
\overline{AB}=14 ,
\overline{BC}=9 ,
\overline{CD}=8 ,
\overline{DA}=12 . What is the radius of the largest possible circle that fits inside or on the boundary of such a quadrilateral?
(2011AMC12A，
https://artofproblemsolving.com/ ... Problems/Problem_24)
(2011中文版AMC12，
https://math.pro/db/thread-1080-1-1.html)
112.6.13補充
若四邊形
ABCD中，
\overline{AB}=8、
\overline{BC}=15、
\overline{CD}=17、
\overline{DA}=10，則四邊形
ABCD的內切圓面積的最大值為
。
(112大直高中，
https://math.pro/db/thread-3759-1-1.html)
計算題2.
設
f(x)=a_nx^n+a_{n-1}x^{n-1}+...+a_1x+a_0 \in Z[x] ，若
a_n ，
a_0 ，
f(1) 均為奇數，試證：方程式
f(x)=0 沒有有理根
(88台中一中高一期末考試題，h ttp://web.tcfsh.tc.edu.tw/jflai/math5/rc/T88113.pdf 連結已失效)
