其他討論請見
http://www.shiner.idv.tw/teachers/viewtopic.php?t=1535
計算題
1.求
tan−131+tan−151+tan−171+tan−181之值為何?
類似題
試求正整數n使得下式成立
tan−131+tan−141+tan−151+tan−1n1=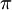 4
4。
(99東山高中,
https://math.pro/db/thread-941-1-1.html)
Find the value of
10cot(cot−13+cot−17+cot−113+cot−121).
(1984AIME,
https://artofproblemsolving.com/ ... Problems/Problem_13)
8.令f(x)為領導係數為1的實係數四次多項式,且
f(99)=2,
f(98)=5,
f(97)=10,
f(96)=17,試求
f(100)=?
2010.7.20
原本的解法有錯,感謝johncai指正
f(x)為四次多項式,且
f(1996)=0,
f(1998)=1,
f(2000)=4,
f(2002)=27,
f(2004)=256,求
f(2008)之值?
(97中一中,
http://forum.nta.org.tw/examservice/showthread.php?t=46779)
若五次實係數多項式f滿足
f(0)=1,
f(1)=3,
f(2)=4,
f(3)=5,
f(4)=6,
f(5)=18,則
f(6)=?
(2004TRML個人賽)
103.9.12補充
計算題9.
今一單位球(半徑為1的球)球心為原點,且球面上兩點P、Q座標分別為
P(1 0
0 0)
0)、
Q(0 2
2 2
2 2
2 2)
2) ,延著球面行進,於PQ最短路徑中取一點R,使得(PR弧長):(QR弧長)=1:2,試求R點座標。

 ∠POQ=90
∠POQ=90
,(PR弧長):(QR弧長)=1:2得到
∠PAR=30
,
∠QOR=60
。
∠PAR=30
,
OR=1,
OA⊥RA得到
OA=2 3
3 ,
AR=21。
OQ =Q
=Q Q=2
Q=2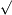 2
2 得到
∠QOQ =45
=45
所以平面POQR和
xy平面夾角
45
,得到
∠RAR =45
=45 AR=21
AR=21,
∠RAR =45
=45
得到
AR =R
=R R=4
R=4 2
2
R點坐標為
(2 3
3 4
4 2
2 4
4 2)
2)
[延伸思考]
假設地球為一球體,今以地球球心為原點,地球半徑為單位長,建立一直角坐標系。設地球表面上有甲乙丙三地,甲、乙兩地的坐標分別為
(1 0
0 0)
0)、
(21 21
21 2
2 2)
2) ,而丙地正好是甲地之間最短路徑的中點,則丙地的坐標為?
(90自然組大學聯考)
(98嘉義高中則將坐標改為
(1 0
0 0)
0)、
(73 72
72 76)
76)其他則一模一樣)
有一種算法是先求甲
(1 0
0 0)
0)、乙
(21 21
21 2
2 2)
2) 的中點
(43 41
41 4
4 2)
2)
長度

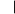
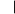
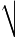
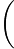 43
43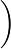 2+
2+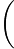 41
41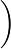 2+
2+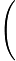 4
4 2
2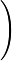 2=42
2=42 3
3
再換成單位向量
142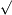 3(43
3(43 41
41 4
4 2)=(2
2)=(2 3
3 6
6 3
3 6
6 6)
6)就是丙地坐標
想想看為什麼可以這樣算?若將條件換成弧長
1:2時,用內分點公式算出三等分點後換成單位向量答案卻是錯的,為什麼這個方法不能用在弧長
1:2的條件上?
99大安高工這題
P(1 0
0 0)
0)在x軸上,
Q(0 2
2 2
2 2
2 2)
2) 在
yz平面上,而且角度有
90
、
45
、
30
等特別角,似乎條件要特別湊好才能算出答案,假若下次考試時P、Q隨便取兩個坐標
(1 14
14 2
2 14
14 3
3 14)
14)、
(32 31
31 32)
32),那你要用什麼方法算出答案呢?
110.8.15補充
今一單位球(半徑為1的球)球心為原點,且球面上兩點
P、
Q座標分別為
P(1 0
0 0)
0) Q(−21
Q(−21 43
43 4
4 3)
3) ,沿著球面行進,於
PQ最短路徑中取一點
R,使得弧
PR:弧
QR=1:3,試求
R點座標。
(1092中山大學雙週一題第6題,
http://www.math.nsysu.edu.tw/~problem/2021s/1092Q&A.htm)
112.4.24補充
將地球儀設定成一個坐標空間,其中球心為原點
O,地球儀上
A,
B兩個城市的坐標分別為
A(1 0
0 0)
0),
B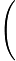 21
21 21
21 2
2 2
2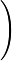
,而
C城市正好是
A,
B兩個城市之間最短路徑的中點,試求
C城市的坐標為?
(112台南女中,
https://math.pro/db/thread-3730-1-1.html)