剛剛發現，第二十題 應該是出自於 AIME 1985
https://artofproblemsolving.com/ ... Problems/Problem_15

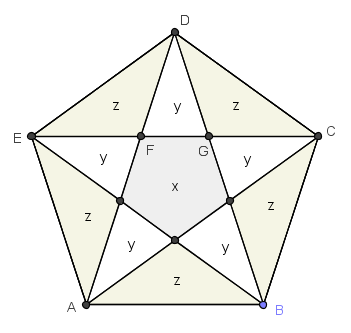
第11題
令\(\displaystyle \phi = \frac{1+\sqrt{5}}{2} \)，易知\(\phi\)滿足方程式\( \phi^2-\phi-1=0\)
不難說明\( \triangle CDF\)、\(\triangle DFG\)、\(\triangle GCD\)皆為頂角\( 36^\circ \)的等腰三角形或頂角\(108^\circ\)的等腰三角形，故皆為黃金三角形
\(\displaystyle \frac{DG}{FG}=\frac{CD}{GD}= \frac{CG}{FG}=\phi \)
\(\displaystyle CD = GD \times \phi = FG \times \phi^2 \)
兩個正五邊形邊長比為 \( \phi^2 \)，故面積比為 \( \phi^4 \)
\(\displaystyle x+5y+5z = x \times \phi^4 = \phi^4 = ( \phi + 1)^2 = \phi^2 +2\phi+1 =3\phi +2 = \frac{7+3\sqrt{5}}{2} \)
\(\displaystyle y:z =FG:CG =1:\phi\)
\(\displaystyle\phi^4 = x +5y +5z = 1+5y +5\phi y\)
\(\displaystyle y = \frac{\phi^4 -1}{5(1+\phi)} = \frac{(\phi^2+1)(\phi+1)(\phi-1)}{5(\phi+1)} = \frac{(\phi+2)(\phi-1)}{5}=\frac{\phi^2+\phi-2}{5} = \frac{2\phi-1}{5}=\frac{\sqrt{5}}{5} \)