引用:
原帖由 bombwemg 於 2011-6-10 05:19 PM 發表 
不好意思~想請問第9,12,13,16(我覺得是對的不知有沒有錯)
12.
設
(1+ 2)n=an+bn
2)n=an+bn 2
2 ,其中
n an
an bn
bn皆為正整數,則
limn
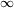 bnan=
bnan= 。
[解答]
因為(1+2^0.5)^n=a_n +b_n *2^0.5 ------------(1)
由二項式定理可知
(1-2^0.5)^n=a_n -b_n *2^0.5 -------------(2)
[(1)+(2)]/2 得a_n= [(1+2^0.5)^n+(1-2^0.5)^n]/2
[(1)- (2)]/2 得b_n = [(1+2^0.5)^n-(1-2^0.5)^n]/(2*2^0.5)
limit {n->infinity} a_n/b_n
=limit {n->infinity} 2^0.5*[(1+2^0.5)^n+(1-2^0.5)^n]/ [(1+2^0.5)^n-(1-2^0.5)^n]
=2^0.5