113中山女高

1.
若f(n)=(n2−2n+1)31+(n2−1)31+(n2+2n+1)31，求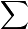500k=11f(2k−1)= 　　　。
(我的教甄準備之路　裂項相消，https://math.pro/db/viewthread.php?tid=661&page=2#pid1678)
連結有解答，https://math.pro/db/viewthread.php?tid=2208&page=1#pid12872

3.
已知ABCD為正四面體，有一隻小蟲反覆在四個頂點之間移動，它從一個頂點爬行至另一頂點稱為一次，已知小蟲從一頂點爬行到任一相鄰頂點的機率均相同，今小蟲從A點開始出發沿稜線爬行至B、C、D其中一點，設an表示小蟲爬行n次後在A點的機率。可以寫出一般式an=　　　。
連結有解答https://math.pro/db/viewthread.php?tid=1318&page=4#pid5071

5.
在坐標平面上，考慮二階方陣A=5134−43 所定義的線性變換。對於平面上異於原點O的點P1，設P1經A變換成P2，P2經A變換成P3。假設P1是圖形y=110x2−10上的動點，則P1P2P3面積的最小可能值為　　　。

在坐標平面上，考慮二階方陣A=5143−34 所定義的線性變換。對於平面上異於原點O的點P1，設P1經A變換成P2，P2經A變換成P3。令a=\overline{OP_1}。
(1)試求sin(\angle P_1OP_3)。
(2)試以a表示\Delta P_1P_2P_3的面積。
(3)假設P_1是圖形\displaystyle y=\frac{1}{10}x^2-10上的動點，試求\Delta P_1P_2P_3面積的最小可能值。
106數甲

6.
卡當諾(Girolamo Cardano,1501-1576)所著的《偉大的技藝(Ars Magna，英譯為The Great art)》書中有一題有趣的三角形面積問題：
There is a triangle the difference between the first and the second sides of which is 1 and between the second and third sides of which is also 1, and the area of which is 3. What is the largest side length? Ans：_______。
(我的教甄準備之路　三角形的面積，https://math.pro/db/viewthread.php?tid=661&page=2#pid2779)
[解答]
設三邊為a=x-1,b=x,c=x+1，最大邊長為c=x+1
利用海龍公式\displaystyle s=\frac{a+b+c}{2}=\frac{3x}{2}
\displaystyle \sqrt{\frac{3x}{2}\left(\frac{3x}{2}-(x-1)\right)\left(\frac{3x}{2}-x\right)\left(\frac{3x}{2}-(x+1)\right)}=3
\displaystyle \frac{3x}{2}\cdot \frac{x+2}{2}\cdot \frac{x}{2}\cdot \frac{x-2}{2}=9
\displaystyle x^2(x^2-4)=48
x^4-4x^2-48=0
\displaystyle x^2=\frac{4\pm \sqrt{4^2+4\cdot 1 \cdot 48}}{2}=\frac{4\pm 4\sqrt{13}}{2}=2\pm 2\sqrt{13}(負不合)
x=\sqrt{2+2\sqrt{13}}
最大邊長c=x+1=\sqrt{2+2\sqrt{13}}+1

10.
已知\displaystyle tan10\theta=\frac{a_1\cdot tan\theta+a_3\cdot tan^3\theta+a_5\cdot tan^5\theta+a_7\cdot tan^7\theta+a_9\cdot tan^9 \theta}{a_0+a_2\cdot tan^2\theta+a_4\cdot tan^4\theta+a_6\cdot tan^6\theta+a_8\cdot tan^8\theta+a_{10}\cdot tan^{10}\theta}，則\displaystyle \sum_{k=0}^{10}|\;a_k|\;=　　　。
相關問題https://math.pro/db/viewthread.php?tid=3350&page=1#pid21520