1.將5個A、5個B及5個C等15個字母排成一列,使得前5個字母沒有A,中間5個字母沒有B,且最後5個字母沒有C,試問共有多少種可能的排列情形。
(2003AMC12,
https://math.pro/db/viewthread.php?tid=454 )
4.設
x y
y R
R,且
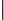 x
x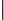
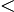 1
1,
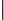 x−y1−xy
x−y1−xy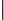
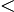 1
1,試求y之範圍?
(75日大自然組,高中數學101 P26,高中數學101修訂版 P26)
9.如圖所示,△ABE中,
∠BAE=90o,C、D為邊
BE上的三等分點,令
BC=CD=DE=a,
AC=7,
AD=9,求a?
(高中數學101 P129,高中數學101修訂版 P130)
類題
三角形ABC中,
∠C=90o,D、E為
AB之三等分點,且
CD=sinX,
CE=cosX,
0o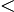 X
X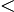 90o
90o,
AB=?
(97全國高中聯招,
http://forum.nta.org.tw/examservice/showthread.php?t=48958)
10.
如下圖,四邊形ABCD中,
AB
 CD
CD且
BC=AD,又
AC及
BD的交點為P,設
BP,
CP,
AD的中點依次為X,Y,Z,且△APB為正三角形,試證△XYZ為正三角形。
(91北一女數學競賽)
http://www.fg.tp.edu.tw/~math/exam/group2/pdf2/921-1a.pdf
我將題目重新打字,方便網友列印
OpenOffice或LibreOffice才能開啟
[
本帖最後由 bugmens 於 2011-5-31 06:30 AM 編輯 ]